Production rate calibration for cosmogenic ¹⁰Be in pyroxene by applying a rapid fusion method to ¹⁰Be-saturated samples from the Transantarctic Mountains, Antarctica

Measurements of multiple cosmogenic nuclides in a single sample are valuable for various applications of cosmogenic nuclide exposure dating and allow for correcting exposure ages for surface weathering and erosion and establishing exposure–burial history. Here we provide advances in the measurement of cosmogenic ¹⁰Be in pyroxene and constraints on the production rate that provide new opportunities for measurements of multi-nuclide systems, such as ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{3}}\mathrm{He}$, in pyroxene-bearing samples. We extracted and measured cosmogenic ¹⁰Be in pyroxene from two sets of Ferrar Dolerite samples collected from the Transantarctic Mountains in Antarctica. One set of samples has ¹⁰Be concentrations close to saturation, which allows for the production rate calibration of ¹⁰Be in pyroxene by assuming production–decay equilibrium. The other set of samples, which has a more recent exposure history, is used to determine if a rapid fusion method can be successfully applied to samples with Holocene to Last Glacial Maximum exposure ages. From measured ¹⁰Be concentrations in the near-saturation sample set we find the production rate of ¹⁰Be in pyroxene to be 3.74 ± 0.10 atoms g⁻¹ yr⁻¹, which is consistent with ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{3}}\mathrm{He}$ paired nuclide ratios from samples assumed to have simple exposure. Given the high ¹⁰Be concentration measured in this sample set, a sample mass of ∼ 0.5 g of pyroxene is sufficient for the extraction of cosmogenic ¹⁰Be from pyroxene using a rapid fusion method. However, for the set of samples that have low ¹⁰Be concentrations, measured concentrations were higher than expected. We attribute spuriously high ¹⁰Be concentrations to failure in removing all meteoric ¹⁰Be and/or a highly variable and poorly quantified procedural blank background correction.

1 Introduction

This paper describes advances in the measurement and application of cosmogenic ¹⁰Be in pyroxene, including a rapid fusion extraction method and a production rate calibration data set. This is important because measurements of multiple cosmogenic nuclides in single samples are valuable for various applications of exposure dating. Multiple-nuclide systematics are useful for correcting exposure ages for surface weathering and erosion (Klein et al., 1986; Nishiizumi et al., 1986; Lal, 1991) and quantifying when and how often a surface has experienced burial (Granger and Muzikar, 2001; Granger, 2006; Balco and Rovey, 2008). For quartz-rich samples, multiple-nuclide measurements (${}^{\mathrm{26}}\mathrm{Al}{/}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{21}}\mathrm{Ne}$) in quartz are common practice and well-established (e.g., Balco and Shuster, 2009). However, multiple-nuclide measurements are generally not feasible in minerals other than quartz.

The stable cosmogenic nuclide ³He is most commonly used in mafic rocks for exposure dating, as it is retentive in both pyroxene and olivine (Blard, 2021) and easily measured using a noble gas mass spectrometer (Balter-Kennedy et al., 2020). Measurements of cosmogenic ¹⁰Be in pyroxene are potentially useful for exposure age applications and have been investigated in prior studies (Balter-Kennedy et al., 2023; Blard et al., 2008; Collins, 2015; Eaves et al., 2018; Ivy-Ochs et al., 1998; Nishiizumi et al., 1990). To fully utilize paired ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{3}}\mathrm{He}$ in pyroxene, it is necessary to constrain the production rate of cosmogenic ¹⁰Be in this mineral.

Cosmogenic nuclide production rates can be quantified in samples by (i) constraining the exposure age by independent radiocarbon and/or other geological dating methods (e.g., Borchers et al., 2016; Blard et al., 2008; Eaves et al., 2018), (ii) measuring the ratio of one nuclide to another with an already well-known production rate (e.g., Niedermann et al., 2007; Luna et al., 2018), and/or (iii) measuring nuclide concentration in samples experiencing negligible erosion rates and having reached production–decay equilibrium (Borchers et al., 2016; Jull et al., 1989; Nishiizumi et al., 1986). In this study, we take advantage of some of the longest exposed rocks in central Antarctica, where erosion rates are negligible, and ³He exposure ages exceeding 8 Ma require that ¹⁰Be concentrations must be close to the production–decay equilibrium (Balter-Kennedy et al., 2020). This provides an opportunity to validate the previously suggested ¹⁰Be production rate in pyroxene constrained by the different approaches described above.

Previously, extraction of ¹⁰Be from pyroxene (e.g., Balter-Kennedy et al., 2023; Blard et al., 2008; Collins, 2015; Eaves et al., 2018) has used wet chemical dissolution and column chromatography similar to that for extracting ¹⁰Be from quartz (Corbett et al., 2016). However, this process is challenging because of the large cation load and the extremely high selectivity required in the column separation. We adopt a ¹⁰Be extraction method involving a total rapid fusion of the pyroxene sample (Stone, 1998) to improve the efficiency of ¹⁰Be extraction from pyroxene. This method is commonly used to extract meteoric ¹⁰Be from a variety of geologic matrices and should therefore be applicable for pyroxene despite the high concentrations of other cations.

We apply the fusion method to two sets of samples. First, we analyze a set of samples with extremely high ¹⁰Be concentrations (10⁷ atoms g⁻¹) that, as described above, can be used for production rate calibration by assuming production–decay equilibrium. Second, we analyze an additional set of samples with much lower ¹⁰Be concentrations (10⁴–10⁵ atoms g⁻¹) to determine if the fusion method can be successfully applied to samples with Holocene to Last Glacial Maximum exposure ages and much lower ¹⁰Be concentrations.

2 Method

2.1 Geological setting and samples

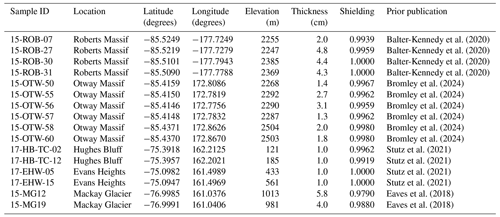
We selected two sets of samples of Ferrar Dolerite from the Transantarctic Mountains (TAM). The Ferrar Dolerite (Harvey, 2001) is a mafic intrusive rock consisting primarily of calcic plagioclase and several orthopyroxenes and clinopyroxenes (Elliot and Fleming, 2021). The first set consists of 10 samples from high elevations in the central TAM that had previous ³He measurements indicating exposure ages >8 Ma. These samples are surface boulders collected from various moraines from Roberts Massif described by Balter-Kennedy et al. (2020) and several similar samples from nearby Otway Massif (Bromley et al., 2024) (Table 1). Erosion rates for Ferrar Dolerite in Antarctica are 0–35 cm Myr⁻¹ (Balter-Kennedy et al., 2023). However, the ³He exposure ages limit the erosion rates for these specific samples to be <5 cm Myr⁻¹, and therefore this set of samples can be expected to have reached production–decay equilibrium (“saturation”) for ¹⁰Be, such that $N_{\mathrm{10}}=P_{\mathrm{10}}/{\mathit{\lambda }}_{\mathrm{10}}$, where N₁₀ is the ¹⁰Be concentration (atoms g⁻¹), P₁₀ is the ¹⁰Be production rate in the sample (atoms g⁻¹ yr⁻¹), and λ₁₀ is the ¹⁰Be decay constant (4.99 × 10⁻⁷ yr⁻¹). After 8 Ma of exposure, ¹⁰Be concentrations have reached 98 % of saturation values. Thus, these samples are expected to have extremely high ¹⁰Be concentrations, facilitating precise measurements. Measuring ¹⁰Be in these samples allows a straightforward estimate of the ¹⁰Be production rate in pyroxene integrated over the last 8 Ma.

The second set of samples is designed to test whether or not the fusion extraction method is also effective for samples with lower ¹⁰Be concentrations. The samples we analyze are low-elevation glacially transported erratics near outlet glaciers of the East Antarctic Ice Sheet in northern Victoria Land. Exposure age chronologies using ¹⁰Be in quartz or ³He in pyroxene from the same sites indicate that these samples have exposure ages of the last glacial–interglacial cycle. In addition, ¹⁰Be in pyroxene was previously measured in two of these samples (MG-12 and MG-19) using a dissolution–cation exchange method by Eaves et al. (2018). We selected this set of samples in part because they had been analyzed for ³He in previous studies (Table 1). We made several additional ³He measurements so that the entire sample set now has both ³He and ¹⁰Be data. The ³He data provide a means of evaluating the accuracy of the ¹⁰Be measurements. Details of the previously analyzed samples are from Stutz et al. (2021) and Eaves et al. (2018) and are summarized in Table 1.

Table 1 Location and site information for samples of Ferrar Dolerite analyzed in this study.

2.2 Mineral separation

The samples were crushed and sieved to a grain size of 75–125 µm at which mostly monomineralic grains were observed. The samples were washed in water and then leached in 10 % HCl at room temperature overnight. We then ran the sample through a magnetic separator to separate pyroxene from the less magnetic plagioclase and other minerals present.

At the University of Vermont/National Science Foundation Community Cosmogenic Facility (CCF), the pyroxene grains underwent hydrofluoric acid (HF) leaching, following Balter-Kennedy et al. (2023), to remove meteoric ¹⁰Be and any plagioclase attached to the pyroxene grains. A fine grain size reduces the amount of meteoric ¹⁰Be stored in the grain fractures, and HF etching was found to be sufficient to remove meteoric ¹⁰Be by Balter-Kennedy et al. (2023), without powdering the sample as otherwise previously suggested (Blard et al., 2008). The samples were leached in HF twice; first in a 1 L solution of 1 % HF in an ultrasonic bath at ∼ 60 °C for 6 h and then again in 1 L of 1 % HF and 1 % HNO₃ overnight, targeting a 20 %–30 % mass loss. During HF leaching, precipitates of fluoride (MgF₂, CaF₂) are produced and are insoluble in dilute HF. Therefore, we did a final leaching in 0.5 % HNO₃ overnight in a heated ultrasonic bath to dissolve the fluoride precipitates.

2.3 Extraction and analyses of cosmogenic ¹⁰Be in pyroxene

The extraction of Be was done at the CCF by total fusion in a potassium bifluoride (KHF₂) flux according to Stone (1998). Samples were processed in two separate batches; the first batch contained the high-concentration samples, and the second batch contained the low-concentration samples. The pure pyroxene samples were powdered using a shatterbox, and 0.5 g of powdered sample was massed into 30 mL platinum crucibles. The sample mass is determined by the size of the Pt crucibles and other properties of the heating apparatus and is chosen to avoid spattering and sample loss during fusion. For the set of samples with expected high ¹⁰Be concentration, we added 400 µg of ⁹Be carrier to each 0.5 g sample. This ⁹Be carrier is a beryl carrier (termed Carrier C) made at the facility with a concentration of 348 µg mL⁻¹. After drying the sample and carrier mixture, anhydrous KHF₂ and anhydrous Na₂SO₄ were added at the ratio of $\mathrm{8}:\mathrm{1}:\mathrm{2}$ ${\mathrm{KHF}}_{\mathrm{2}}:{\mathrm{Na}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}:\mathrm{sample}$ by weight to the crucibles and homogenized.

The fusion protocol at the CCF uses 30 mL platinum crucibles. While it is possible to fuse larger (1–2 g) samples in larger (100 mL) crucibles (Stone, 1998), these are not compatible with the fixed fluxing apparatus used to minimize the hazard of molten KHF₂. To increase the sample size and the measured ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{9}}\mathrm{Be}$ ratio for the set of expected low ¹⁰Be concentration samples, we fused 1 g of sample in two separate fusions of 0.5 g each, with half as much carrier (200 µg) as used for the initial sample batch. With sample and carrier concentrations similar in both aliquots (specifically, as close as possible with the weighing and dispensing equipment in use; we estimate better than 1 % agreement between aliquots), ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{9}}\mathrm{Be}$ ratios in both aliquots after fusion can be expected to be identical, so we combined them to yield a higher sample/carrier ratio than possible in a single fusion.

Before starting this procedure, we determined whether halving the amount of ⁹Be carrier would affect the Be yield by fusing aliquots of sample 15-OTW-60 with varying amounts of added ⁹Be carrier. The ⁹Be yields were measured by inductively coupled plasma optical emission spectroscopy (ICP-OES) at the CCF. Total ⁹Be yields (Table 2) show that less ⁹Be does not result in a lower Be yield. Because Be yields in the first set of high-concentration samples were lower than expected, we increased the amount of Na₂SO₄ added to a ratio of $\mathrm{4}:\mathrm{2}:\mathrm{1}$ ${\mathrm{KHF}}_{\mathrm{2}}:{\mathrm{Na}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}:\mathrm{sample}$ by weight as suggested for calcium-rich samples by Stone (1998). This change makes sense because the Ferrar pyroxene is calcic, and having an abundance of SO₄ during fluxing suppresses the formation of CaBeF₄, which is less soluble. This modification significantly increased the total Be yield (Table 2).

After fusion, the Stone (1998) procedure involves Be and K extraction by water leaching and removal of residual fluorides by centrifuging as BeF₂ is soluble and most other fluorides are not. The two aliquots of each sample were combined, and K was removed from the combined sample by precipitation of KClO₄. The supernatant was evaporated to remove the remaining HClO₄ and redissolved in 12 mL of dilute HNO₃. At this point, we experienced difficulty in completely redissolving the precipitated sample and found it necessary to centrifuge the sample multiple times to remove what we presumed to be the remaining KClO₄. Although Be yields from the samples that have low ¹⁰Be concentrations were as expected (Balco et al., 2021), the resulting accelerator mass spectrometry (AMS) targets had unusually low beam currents, which made AMS measurement more difficult than expected (Table 2). We hypothesize that this is most likely the result of K carryover in the final stages of the extraction process and that this could have been prevented by increasing the volume of the final HNO₃ solutions to dissolve K more effectively.

Ratios of ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{9}}\mathrm{Be}$ were measured at Lawrence Livermore National Laboratory (LLNL) and normalized to the 07KNSTD3110 standard (Nishiizumi et al., 2007) with a ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{9}}\mathrm{Be}$ ratio of 2.85 × 10⁻¹². Uncertainties in calculated ¹⁰Be concentrations include AMS measurement uncertainties, uncertainty in the Be carrier concentration, and uncertainty in blank corrections (Table 2). Five procedural blanks measured with both sample batches had a mean and standard deviation of 128 000 ± 67 000 atoms ¹⁰Be. This is less than 0.4 % of the total amount of ¹⁰Be measured in any of the samples in the high-concentration batch (Table 2), so blank correction uncertainty makes a negligible contribution to overall measurement uncertainty for these samples. However, the highest blank values were up to 60 % of the total number of atoms measured in some of the low-concentration samples, so blank uncertainty is significant for the low-concentration batch. We discuss this in more detail in Sect. 3.5.

2.4 Cosmogenic ³He analysis

We measured cosmogenic ³He concentrations in all samples at Berkeley Geochronology Center (BGC) following the procedure described in Balter-Kennedy et al. (2020). ³He concentrations for two samples, HB-TC-02 and HB-TC-12, have already been reported in Stutz et al. (2021). Measurements of the CRONUS-P intercomparison standard (Blard et al., 2015) during the period of these measurements were 5.03 ± 0.15 × 10⁹ atoms g^{−1 3}He (Balter-Kennedy et al., 2020), which is indistinguishable from the accepted value of 5.02 ± 0.12 × 10⁹ atoms g⁻¹ (Blard et al., 2015).

3 Results and discussion

3.1 Measured cosmogenic ¹⁰Be in saturated samples

Measured ¹⁰Be concentrations in the set of high-concentration samples range from 5.92–7.67 × 10⁷ atoms g⁻¹ with uncertainties <2.2 % (Tables 2 and 3). These are equivalent to some of the highest in situ ¹⁰Be concentrations measured in terrestrial rocks (Spector and Balco, 2020). As expected from the elevation dependence of the ¹⁰Be production rate and the assumption that the ¹⁰Be concentrations are close to production–decay saturation, the measured concentrations increase systematically with elevation (Fig. 1).

Table 2 Measured Be results, including yields measured by ICP-OES in the dilute HNO₃ solution prior to final precipitation, with implied Be yields for the fusion process and measured AMS currents and ratios.

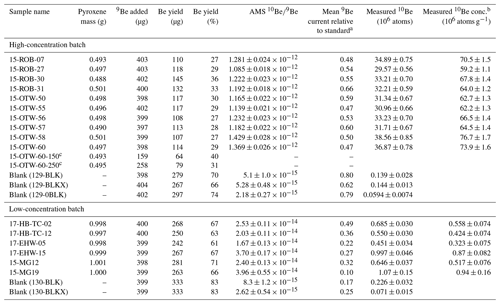
^a Mean current for the KNSTD3110 is 21.5 µA. ^b The measured ¹⁰Be concentrations include blank correction. ^c Samples were processed only as a yield test, and no AMS measurements were made.

Table 3 The ³He and ¹⁰Be concentrations for long-exposure glacial erratics in the Transantarctic Mountains. The ¹⁰Be production rate is determined from Eq. (1).

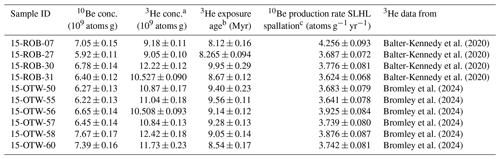
^a For samples where more than one measurement exist the concentration represents the error-weighted mean and the standard error. ^b The uncertainty in the age is the internal uncertainty using the online exposure age calculator. ^c The sea level/high-latitude (SLHL) reference ¹⁰Be production rate is determined from Eq. (1) and the scaling method of Stone (2000), as implemented in Balco et al. (2008).

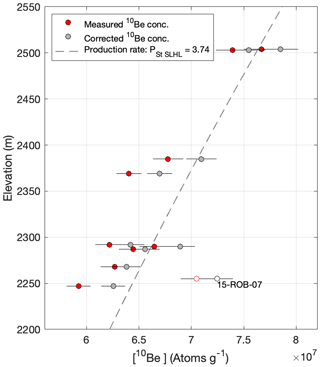
Figure 1 Measured ¹⁰Be concentrations vs. elevation. Red dots are measured ¹⁰Be concentrations as reported in Table 3, gray dots show measured ¹⁰Be concentrations corrected for sample thickness and shielding, and the dashed line shows the saturated ¹⁰Be concentrations for the “St” reference production rate of 3.74 atoms g⁻¹ yr^{−1 10}Be in pyroxene. White dots indicate sample outlier, which is not included in the production rate calibration (see Sect. 3.2).

3.2 The ¹⁰Be production rate in pyroxene

In general, as discussed above, ³He exposure ages range between 8–10 Ma (5–6 times the ¹⁰Be half-life) and imply that ¹⁰Be concentrations in these samples are within 1 %–2 % of production–decay saturation. We account for the small, predicted difference from the saturation concentration by calculating the production rate as follows:

$$\begin{array}{}\text{(1)}& P_{\mathrm{10}}={\displaystyle \frac{N_{\mathrm{10}}{\mathit{\lambda }}_{\mathrm{10}}}{\left(\mathrm{1}-e^{-{\mathit{\lambda }}_{\mathrm{10}}{t}_{\mathrm{3}}}\right)}},\end{array}$$

where P₁₀ is the ¹⁰Be production rate in the sample (atoms g⁻¹ yr⁻¹), N₁₀ is the ¹⁰Be concentration (atoms g⁻¹), λ₁₀ is the ¹⁰Be decay constant (4.99 × 10⁻⁷ yr⁻¹), and t₃ is the ³He exposure age (yr). Because the samples are close to production–decay saturation, the production rate determined from Eq. (1) is insensitive to uncertainty in the assumed exposure age. Therefore, although we use the apparent ³He exposure ages to correct for an inferred small systematic difference from production–decay saturation, the accuracy of the ³He ages is minimally important for the ¹⁰Be production rate estimate. To obtain the spallogenic production rate of ¹⁰Be in pyroxene, we subtract the production rate in pyroxene due to muons using the muon interaction cross-sections of Balter-Kennedy et al. (2023), which account for <1 % of the production rate, and correct for sample thickness and topographic shielding.

Applying the “St” elevation scaling of Stone (2000) then yields sea level/high-latitude (SLHL) production rates in the range of 3.6–4.3 atoms g⁻¹ yr⁻¹ (Table 3). The ¹⁰Be production rate increases with elevation, so samples near or at saturation are expected to likewise have ¹⁰Be concentrations increasing with elevation. This is true for all samples, except 15-ROB-07, which has an excess ¹⁰Be concentration equivalent to ∼ 250 m (Fig. 1). Removing one outlier (15-ROB-07; see Fig. 1) yields a mean and standard error of 3.74 ± 0.10 atoms g⁻¹ yr⁻¹.

The production rate estimate agrees with that of Balter-Kennedy et al. (2023) (3.6 ± 0.2 atoms g⁻¹ yr⁻¹), which was cross-calibrated with the ³He production rate. However, in the present study, our calibration is independent of the ³He production rate, where samples with near-saturated ¹⁰Be concentrations permit a direct calculation of the production rate from the measurements. In contrast, the sample set in the Balter-Kennedy et al. (2023) study lacks direct constraints on the exposure age and/or exposure history, and a best-fit production rate was computed from values that permitted all the samples to have a simple exposure history bounded by limiting assumptions of steady exposure at zero erosion and steady erosion for an infinite time. While they are not directly comparable, it is possible to determine whether the two data sets are consistent with each other and with the assumption of simple exposure. In Fig. 2 we construct a ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{3}}\mathrm{He}$ two-nuclide diagram using the production rate determined from our study and an assumed ³He production rate of 120 atoms g⁻¹ yr⁻¹ (Borchers et al., 2016), and plot the ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{3}}\mathrm{He}$ data from both studies. This shows that all data from both studies (except for one outlier in our study identified above) plot within the simple exposure region and are therefore internally consistent.

Figure 2 A ¹⁰Be-³He two-nuclide diagram. Red data points show measurements from this study, green data points are from Balter-Kennedy et al. (2023), and each shaded ellipse represents the 68 % confidence interval in the measured nuclide concentrations. The thick blue line is the simple exposure line, and the thin blue lines are lines of constant erosion (m Myr⁻¹). The thick black line is the steady-erosion line, and the thin black lines are constant-age lines (Myr). ^* signifies nuclide concentrations normalized to site-specific production rate for comparison across sampling locations.

Finally, we consider whether our data are consistent with other ¹⁰Be-in-pyroxene production rate calibration data and with commonly used production rate scaling methods. Two other studies obtained ¹⁰Be-in-pyroxene production rate calibration data from samples with independent age constraints. Blard et al. (2008) included two samples (SI41 and SI43) from separate lava flows at Mount Etna, Italy, with $\mathrm{K}/\mathrm{Ar}$ ages of 33 and 10 kyr, respectively. Eaves et al. (2018) obtained three samples from the Murimotu formation debris avalanche at Mount Ruapehu, New Zealand, which has a radiocarbon age of 10.5 kyr. In Fig. 3, we apply the production rate calibration code from version 3 of the online exposure age calculator originally described by Balco et al. (2008) and subsequently updated to (i) our production rate calibration data alone and (ii) our data with the Blard et al. (2008) and Eaves et al. (2018) data. One aspect of this comparison is that our data are from relatively high elevations and high latitudes, and the other calibration data are from relatively low elevations and moderate latitudes.

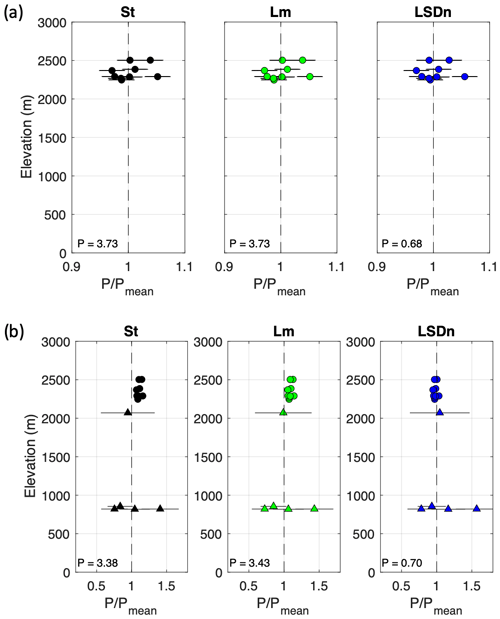
Figure 3 Relative variation with elevation in production rate scaling parameters calculated from calibration samples in this study (high-elevation data; shown in panels a and b as circles) and those of Blard et al. (2008) and Eaves et al. (2018) (lower-elevation data; shown in panel b only as triangles). For the “St” and “Lm” scaling methods, the production rate scaling parameter P is a reference production rate with units of atoms per gram per year; for the “LSDn” scaling method, it is a non-dimensional correction factor. Note that the x-axis limits are different in (a) and (b). The reference production rate (P) value for each scaling factor is the calculated site-weighted mean.

The production rate of ¹⁰Be in pyroxene is predicted to vary with the major element composition of the target mineral. Element-specific production rates calculated by Masarik (2002) predict up to a possible 27 % variation between the extreme end member pyroxene compositions (enstatite vs. ferrosilite). However, the variation among the composition of pyroxene in which ¹⁰Be concentrations have been measured is much less, as the mineral separation process used to prepare samples for ¹⁰Be analysis does not select for individual pyroxenes. Using the element-specific predictions from Masarik, the full range of pyroxene compositions observed in the Ferrar Dolerite (Elliot and Fleming, 2021) predicts a maximum 6.5 % variation in the production rate. Pyroxene compositions in our high-concentration sample set used for production rate calibration (Table 4) predict a maximum production rate difference of 5 % and a standard deviation of 2 % (Table 4). Furthermore, pyroxene compositions in previous production rate calibration studies (Blard et al., 2008; Collins, 2015) fall within the range predicted for Ferrar pyroxenes. Thus, although variations in production rates due to pyroxene composition may be important in some situations, they are likely at the level of measurement uncertainty for available calibration data.

Table 4 Major element composition (oxide wt %) and theoretically simulated SLHL ¹⁰Be production rate in pyroxene.

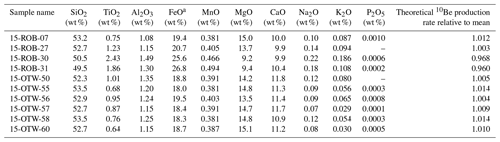
^a Total Fe expressed as FeO. ^b The theoretical production rate is calculated from the Masarik (2002) formula for estimating the compositional dependence of the ¹⁰Be production rate in pyroxene and results in a mean value of 4.55 and standard deviation of 0.09 atoms g⁻¹ yr⁻¹. Note that although the inter-element variation in predicted production rates in this study is expected to be accurate, the absolute value of the production rate (e.g., the value of 4.55 atoms g⁻¹ yr⁻¹) was calculated by reference to obsolete ¹⁰Be measurement standards and is not expected to be accurate.

Taken together, we find that the reference production rate of 3.74 ± 0.10 atoms g⁻¹ yr⁻¹ determined in this study is consistent with other ¹⁰Be-in-pyroxene production rate calibration data (Blard et al., 2008; Eaves et al., 2018) and in agreement with the previously published production rate of 3.6 ± 0.2 atoms g⁻¹ yr⁻¹ (Balter-Kennedy et al., 2023) with an overall improvement in the uncertainty.

3.3 The ¹⁰Be and ³He measurements in low-concentration samples

The ¹⁰Be concentrations from the set of young-exposure-age erratics, as expected, were 2 orders of magnitude lower than concentrations in the high-elevation, saturated samples (Table 5). As discussed above, these samples are glacially transported erratics found near the margins of major glaciers in the Transantarctic Mountains. The geomorphic context, ³He exposure ages on these and nearby samples, and ¹⁰Be exposure ages on nearby quartz-bearing samples, all indicate that these samples were emplaced by deglaciation during the last glacial–interglacial cycle and have most likely not experienced more than 50 000 years of exposure (Stutz et al., 2021; Eaves et al., 2018).

Given the assumptions that (i) the samples have experienced exposure only in the last ∼ 50 000 years and (ii) the non-cosmogenic ³He concentration is constant among samples, measured ³He and ¹⁰Be concentrations should be linearly related, with a slope given by the ${}^{\mathrm{3}}\mathrm{He}{/}^{\mathrm{10}}\mathrm{Be}$ production ratio and an intercept on the ³He axis given by the non-cosmogenic ³He concentration in Ferrar pyroxene. Non-cosmogenic ³He in Ferrar pyroxene is most likely derived from nucleogenic production and has been estimated in various studies to be less than approximately 6 × 10⁶ atoms g⁻¹ (Eaves et al., 2018; Kaplan et al., 2017; Margerison et al., 2005).

Combining our ³He measurements with the ¹⁰Be concentrations obtained from Collins (2015) and Eaves et al. (2018) results in the expected linear relationship, with a slope of ${}^{\mathrm{3}}\mathrm{He}{/}^{\mathrm{10}}\mathrm{Be}$ = 28.5 ± 4.6 and ³He intercept of 3.9 ± 0.8 × 10⁶ atoms g⁻¹ (Fig. 4). If we take the reference ³He production rate to be 120 ± 13 atoms g⁻¹ yr⁻¹, which is derived for “St” scaling with the calibration data set of Borchers et al. (2016), this slope implies a ¹⁰Be production rate of 4.20 ± 0.82 atoms g⁻¹ yr⁻¹, which is consistent with, albeit less precise than, the other estimates discussed in the previous sections. The ³He intercept is most likely a good estimate of the nucleogenic ³He concentration in Ferrar pyroxene.

However, only one of the ¹⁰Be concentrations measured in this study agrees with the expected linear relationship; the others are systematically higher than expected, by hundreds of thousands of atoms per gram. In particular, MG12 and MG19 were measured both by Eaves et al. (2018) and in this study; our results are 3.94 × 10⁵ and 8.4 × 10⁵ atoms g⁻¹ higher than those of Eaves et al. (2018), respectively (Table 5). Two possible explanations for this discrepancy are (i) failure to completely remove meteoric ¹⁰Be_m before extraction or (ii) a highly variable and poorly quantified procedural blank background correction (Table 2). Both scenarios are discussed in the following sections.

Table 5 Measured ³He and ¹⁰Be concentrations in low-concentration samples from glacial transported erratics during the last glacial-interglacial cycle, including published concentrations from others.

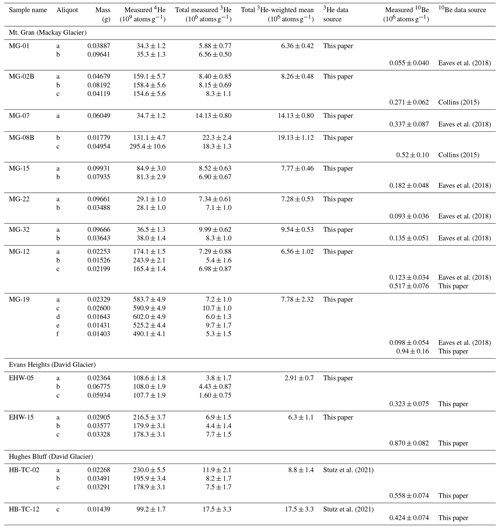
All ³He measurements employed the BGC “Ohio” NGMS system. Analytical methods are as described in Balter-Kennedy et al. (2020). The ¹⁰Be data from Eaves et al. (2018) and Collins (2015) were originally normalized to the NIST SRM4325 standard with an assumed ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{9}}\mathrm{Be}$ ratio of 3 × 10⁻¹¹ and have been renormalized to the “07KNSTD” standardization of Nishiizumi et al. (2007).

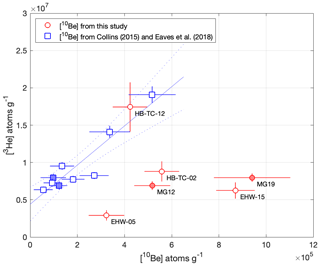
Figure 4 Measured ¹⁰Be and ³He concentrations in low-concentration samples. Red dots are sample data with ¹⁰Be concentrations measured in this study. Blue squares are sample data with ¹⁰Be concentrations obtained from Collins (2015) and Eaves et al. (2018). Solid points represent samples that have duplicated ¹⁰Be measurements from this study and Eaves et al. (2018). The horizontal and vertical lines associated with each data point are the measured uncertainties in the nuclide concentrations. The solid blue line is the York regression linear relationship for the blue data points only with a 95 % confidence bound (dashed blue lines).

3.4 Removal of meteoric ¹⁰Be

Failure to successfully remove all meteoric ¹⁰Be_m during HF etching would result in spuriously high concentrations of presumed cosmogenic ¹⁰Be. Balter-Kennedy et al. (2023) found that when using fine to medium grains of pyroxene (32–125 µm), ∼ 25 % mass loss after leaching a sample in 1 % HF/1 %HNO₃ is sufficient to remove meteoric ¹⁰Be_m. After leaching, we observed 35 %–49 % mass loss, indicating that leaching should have been sufficient. Figure 5 compares the mass lost during HF etching to the residual between the measured and predicted in situ cosmogenic ¹⁰Be concentration (atoms g⁻¹), normalized to the error in measured concentrations and calculated using the production rate from this study of 3.74 atoms g⁻¹ yr⁻¹ and the minimum ³He ages for both the high- and low-concentration samples. We see no clear relationship between mass loss and the ¹⁰Be residual for either of the two sample sets, as expected. This is especially evident in samples HB-TC-12 and MG19, which both display similar mass loss (∼ 48 %).

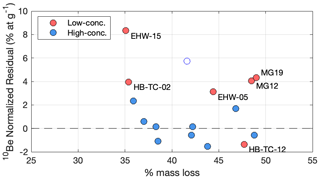
Figure 5 Comparison of mass lost during HF etching with normalized residuals between measured ¹⁰Be concentrations in samples and expected concentrations. For the high-concentration samples (blue), the expected values are given by the dashed line in Fig. 1. For the low-concentration samples (red), the expected values are given by the linear regression in Fig. 4. The white dot represents the outlier as discussed in Fig. 1.

If we were to assume that the increased ¹⁰Be is solely meteoric, then that contributes ∼ 6 × 10⁵ atoms g⁻¹. This is estimated from the average difference between the ¹⁰Be concentrations measured for the replicated samples from this study and those from Eaves et al. (2018), which is assumed to be free of meteoric ¹⁰Be (Table 5). Such contribution would account for less than 1 % of the ¹⁰Be concentration measured for the set of high-concentration samples used for estimating the production rate of ¹⁰Be in pyroxene. Therefore, any potential contribution from meteoric ¹⁰Be would most likely have an insignificant impact on the reference production rate reported in Sect. 3.2.

As dissolved plagioclase attached to pyroxene grains contributes to the total mass loss after leaching, the total mass loss is not a direct reflection of the mass of pyroxene lost that is presumed to contain meteoric ¹⁰Be_m. While the >35 % mass loss is mostly pyroxene, some unknown fraction could be from plagioclase. We can therefore not exclude that samples may contain some meteoric ¹⁰Be_m. However, the lack of correlation between the residuals vs. expected values and the mass loss during etching makes it unlikely that the systematically measured excess in ¹⁰Be concentration is solely caused by meteoric ¹⁰Be_m.

3.5 Uncertainty in the blank correction

The blank correction may be one of the major challenges for analyzing low ¹⁰Be concentration samples, and a highly variable blank could cause a scatter and increase in measured ¹⁰Be concentrations that we observed. The blank correction value is obtained from the average of all five blanks processed during both the high- and low-concentration sample sets. However, the blanks are highly variable between 71 000 and 288 000 ¹⁰Be atoms, which accounts for 10 %–60 % of the total measured ¹⁰Be atoms in the low-concentration batch. If we assume a blank of 71 000 ¹⁰Be atoms for sample HB-TC-02, we get a corrected ¹⁰Be concentration of 6.15 × 10⁵ atoms g⁻¹. However, if we assume a blank of 288 000 ¹⁰Be atoms, we get a ¹⁰Be concentration of 3.97 × 10⁵ atoms g⁻¹, a significantly lower ¹⁰Be concentration. Thus, variability in the measurement background may account for a significant fraction of the difference between measured and expected concentrations. It would only be possible to quantify this contribution of ¹⁰Be by measuring additional blanks and replicates of low-concentration samples.

3.6 Limitations in extracting cosmogenic ¹⁰Be from pyroxene by fusion

Agreement of our production rate estimate from saturated samples with all other existing data shows that extraction of cosmogenic ¹⁰Be from pyroxene by total rapid fusion is effective and accurate for samples with high ¹⁰Be concentrations. Previous studies of ¹⁰Be in pyroxene used wet chemical dissolution and ion exchange chromatography, similar to the procedure used in extracting ¹⁰Be from quartz. However, concentrations of the major cations Ca, Fe, Mg, and Na are much greater in pyroxene than the trace levels found in quartz, which requires substantial scaling up of ion exchange columns (Eaves et al., 2018). The total fusion method of Stone (1998), having extremely high selectivity for Be relative to these cations, completely avoids this issue. However, we were not able to sufficiently scale up the rapid fusion method to obtain the desired signal-to-noise ratio during AMS analysis for the lower-concentration samples.

3.6.1 Sample size limitations

The main obstacle to measuring cosmogenic ¹⁰Be in pyroxene at low concentrations is the difficulty in increasing the sample size to obtain a higher ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{9}}\mathrm{Be}$ ratio and thus signal-to-background ratio. This is a challenge for both extraction methods, albeit for different reasons. For young-exposure-age samples (5–33 kyr), Eaves et al. (2018) dissolved 1.1–2.8 g of pyroxene using large ion exchange columns. For our extraction by total fusion, the sample size is limited to 0.5 g by the size of the Pt crucibles. Note that Stone (1998) processed samples up to 4 g using 100 mL crucibles.

As discussed above, to address the crucible size limitation, we merged duplicate samples of 0.5 g to obtain a total sample mass of 1 g, but increasing the amount of K present in the final steps of the procedure most likely resulted in incomplete separation of K from Be. This, in turn, may have suppressed AMS beam currents (Table 2) and resulted in poor measurement precision for some samples. This could likely be corrected by increasing solution volumes in some steps of the procedure and repeating various precipitation steps to ensure the complete removal of K.

4 Conclusion

In this study, we provide advances in the measurement and application of cosmogenic ¹⁰Be in pyroxene by applying a rapid fusion extraction method (Stone, 1998) and a production rate calibration data set. We extracted and measured cosmogenic ¹⁰Be in pyroxene from two sets of Ferrar Dolerite samples. One set of samples consisting of 10 high-elevation boulders collected from moraines in the upper TAM have ³He measurements indicating that these samples have ¹⁰Be concentration close to saturation. We use this sample set to calibrate the production rate of ¹⁰Be in pyroxene by assuming production–decay equilibrium. The other set of samples consisting of six low-elevation glacially transported erratics from northern Victoria Land are used to test whether or not a rapid fusion extraction method is feasible for samples that have low ¹⁰Be concentrations.

From measured ¹⁰Be concentrations in the near-saturation sample set we find the production rate of ¹⁰Be in pyroxene to be 3.74  ± 0.10 atoms g⁻¹ yr⁻¹, which is in agreement with previously published production rates (Balter-Kennedy et al., 2023; Eaves et al., 2018; Blard et al., 2008), and consistent with ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{3}}\mathrm{He}$ paired nuclide ratios from samples assumed to have simple exposure. Given the high ¹⁰Be concentration measured, a sample mass of ∼ 0.5 g of pyroxene with 400 µg added ⁹Be carrier is sufficient for obtaining meaningful ${}^{\mathrm{10}}\mathrm{Be}{/}^{\mathrm{9}}\mathrm{Be}$ ratios well above blank levels. Even with relatively low Be yields, there is still enough total Be present for AMS detection. Therefore, the extraction of cosmogenic ¹⁰Be from pyroxene samples using rapid fusion works well for samples with high ¹⁰Be concentrations. However, for the sample set that shows low ¹⁰Be concentrations, the measured concentrations are higher than expected by 320 000–810 000 atoms g⁻¹. We attribute this increased ¹⁰Be concentration to potential failure in completely removing all meteoric ¹⁰Be and/or a highly variable and poorly quantified procedural blank background correction.

Advances in measuring ¹⁰Be in pyroxene and constraints on the production rate provide new opportunities for multi-nuclide measurement in pyroxene-bearing samples that allow for correcting exposure ages for surface weathering and erosion and establishing exposure–burial histories.